MAIN PAGE
> Back to contents
Cybernetics and programming
Reference:
Kalabin A.L., Udalov E.V.
The Regression Method of Reducing the Boundary Equation to the Cauchy Problem When Modeling Chemical Fiber Treatment
// Cybernetics and programming.
2016. № 4.
P. 86-90.
DOI: 10.7256/2306-4196.2016.4.19735 URL: https://en.nbpublish.com/library_read_article.php?id=19735
The Regression Method of Reducing the Boundary Equation to the Cauchy Problem When Modeling Chemical Fiber Treatment
Kalabin Aleksandr Leonidovich
Doctor of Physics and Mathematics
professor of the Department of Computer Software at TvSTU (Tver State University)
170026, Russia, Tver Region, Tver, nab. Afanasiya Nikitina, 22

|
kalabin@tstu.tver.ru
|
|
 |
|
Udalov Evgeniy Vadimovich
head of the Division of Enterprise Development at GUTA BANK
170100, Russia, Tver Region, Tver, Tverskoi prospekt, 6, of. 220

|
evgeny.udalov@mail.ru
|
|
 |
|
DOI: 10.7256/2306-4196.2016.4.19735
Received:
13-07-2016
Published:
26-08-2016
Abstract:
The subject of the research is the numerical method implemented in the exploratory research program system applied during technical procedures of chemical fiber treatment. The aforesaid method allows to execute the selection of indeterminate initial conditions based on determinate boundary conditions, i.e. reduce the boundary equation to the Cauchy problem. The method also includes verification of numerical solutions through calculating the initial equation of force balance. This is a task that appears when solving a set of non-linear ordinary differential equations of second order (mathematical model of chemical fiber treatment) by using the 4th-order Runge-Kutta equation. The authors note that it is possible to apply a determinate shooting method together with dichotomy methods and Newton's (tangent) methods. The authors pay attention to the analysis of the shooting method, in particular, describe dependencies of the method iteration number on some initial conditions, of the initial rate - on the finishing fiber speed. The authors also give an insight into the regression method and compare efficiency of the regression method to the shooting method. The authors give a mathematical description of the process of chemical fiber treatment and select a mathematical model. Based on this model, the author carried out a computing experiment using the shooting method. The analysis of the results of the numerical modeling using the shooting method allowed to offer the regression model. The authors also describe drawbacks of using a determinate shooting method reducing the boundary equation to the Cauchy problem when modeling the process of chemical fiber treatment. The authors define regression equations relating a determinate boundary condition and indeterminate initial condition. The authors offer the regression method that converges by approximately 30 % faster than determinate dichotomy and Newton's methods.
Keywords:
mathematical modeling, exploratory research program, shooting method, regression method, Cauchy Problem, boundary equation, numerical method, computing experiment, chemical fibers, treatment modeling
This article written in Russian. You can find original text of the article here
.
Особенности элонгационного течения тонких неизотермических
струй расплавов полимеров заключаются в сопутствующих ему процессах диффузии и
теплопереноса [1]. При охлаждении расплава полимера существенно увеличивается
его вязкость и могут начаться процессы стеклования или кристаллизации.
Увеличение вязкости значительно влияют на характеристики движения. Однако
неравномерность течения при растяжении и изменение радиуса струи влияют на взаимодействие
струи со средой движения и интенсивность протекания всех процессов диффузии и
теплопереноса.
Практической задачей, в которой применяются результаты
изучения элонгационного течения струй расплавов полимеров, является
производство (формование) синтетических волокон [2]. Для использования
математических моделей на практике разработана программная система, так как,
во-первых, процессы формования описываются нелинейными уравнениями с подвижной
границей, требующие численного решения [3,4]; во-вторых, отдельные программные
блоки используются многократно; в-третьих, для структурирования целесообразно
сведение в базу данных исходных данных различных видов формования, используемых
элементов схем технологического процесса, свойств расплавов полимеров.
В гидроаэродинамике используется система уравнений
неразрывности, движения, теплообмена и состояния, которые отражают законы
сохранения массы, импульса и энергии [1]. Отметим некоторые сложности
использования системы общих уравнений для исследования элонгационного течения
струй. Во-первых, настоящий уровень проведения эксперимента не дает возможность
определить величины, входящие в краевые условия, например, распределение
скорости или напряжения по радиусу. Во-вторых, из-за существенной нелинейности
и большой размерности системы затруднена ее параметрическая идентификация.
В-третьих, из-за первой и второй проблем сложно провести достоверное сравнение
результатов расчетов с экспериментом. Наконец, в-четвертых, возникают очевидные
трудности при применении численных методов для решения системы большой
размерности (более 30) с указанными граничными условиями. На данный момент
времени это – не решенная полностью проблема. Для решения указанных проблем
нами предлагается подход, который заключается в исследовании элонгационного
течения струй путем редукции общих уравнений. Редуцированные (упрощенные) системы
уравнений более доступны для решения, анализа, а также для них имеются реальные
экспериментальные данные.
На основе допущений для
описания элонгационного течения тонкой струи из уравнения Навье-Стокса и
краевых условий возможно получить одномерные уравнения движения и теплообмена –систему
обыкновенных дифференциальных уравнений (СОДУ):
движения
v'' +{(μ1(T) – v' /v) + ρ1(v)}v' /μ2(T) +
ρ2(v) /μ2(T)=0 (1)
теплообмена
T‘(x) = – α(v) [T(x) – TS
(x)]. (2)
при
v(0) = v0; v(L) = vL; T(0) = T0. Здесь v(x) – скорость
волокна; T(x) – температура
волокна; v'
= dv/dx
(штрих означает дифференцирование по x); ρi(v), μi(T) и
α(v) –
функции от указанных аргументов.
Основные этапы работы программы моделирования формования
химических волокон с приемным устройством это: ввод начальных условий (параметры
технологического процесса, свойства полимера, свойства расчётной схемы), сплайн-интерполяция
экспериментальных функций, расчёт (подбор начальных условий, решение СОДУ) и
верификация решения.
При численном решении уравнения движения, являющегося обыкновенным
дифференциальным уравнением (ОДУ) II порядка v”(v’, v, x)=0, необходимы начальные условия v(0)и v’(0). Однако из известны лишь граничные значения v(0) и v(L).
Возникает задача перехода от краевой задачи к задаче Коши. Поэтому в работе
будут рассмотрены алгоритмы нахождения v’(0) по известному
значению v(L).
Для численного решения краевых задач применяют метод стрельбы
или иные методы, которые основаны на сведении краевой задачи к некоторой задаче
Коши для той же системы уравнений [5,6].
Метод стрельбы (пристрелки) заключается в использовании
левого краевого условия  и определении значения и определении значения 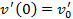 с требуемой точностью по известному левому краевому
условию с требуемой точностью по известному левому краевому
условию 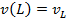 . Эта задача решалась методом дихотомии,
аналогичный решению нелинейных уравнений. Он заключается в следующем. Определяется
диапазон искомого значения . Эта задача решалась методом дихотомии,
аналогичный решению нелинейных уравнений. Он заключается в следующем. Определяется
диапазон искомого значения 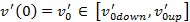 так, что их соответствующие значения
vi(L) меньше и больше заданного
значения v(L)= vL. Пара таких значений образует
«вилку». Деля ее последовательно пополам до получения нужной точности,
производим «пристрелку». Для этого
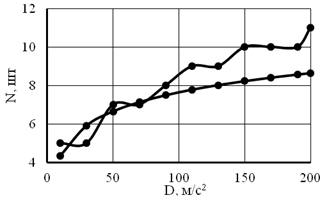
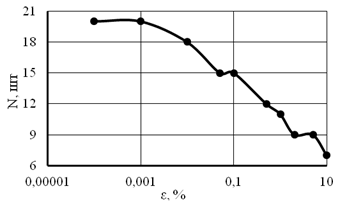
метода определены зависимости количества итераций от величины начального
диапазона искомого значения начального градиента скорости (рис. 1, график 1) и
количества шагов итерации от величины заданной точности поиска финишной
скорости (рис. 2). так, что их соответствующие значения
vi(L) меньше и больше заданного
значения v(L)= vL. Пара таких значений образует
«вилку». Деля ее последовательно пополам до получения нужной точности,
производим «пристрелку». Для этого
метода определены зависимости количества итераций от величины начального
диапазона искомого значения начального градиента скорости (рис. 1, график 1) и
количества шагов итерации от величины заданной точности поиска финишной
скорости (рис. 2).
Можно
представить теоретическую зависимость количество итераций N от величины начального диапазона искомого значения начального
градиента скорости ΔV при заданной абсолютной
погрешности y
в виде
N=log2(ΔV)/v
Моделирование показывает удовлетворительное согласие этой
теоретической зависимости и при
реальном моделировании (рис. 1, график 2).
|
|
|
|
Рис.
1. Зависимость количества итераций от величины начального диапазона искомого
значения начального градиента скорости: 1 – численное моделирование; 2 –
расчет оценки
|
Рис. 2. Зависимость количества итераций от величины
заданной точности поиска финишной скорости
|
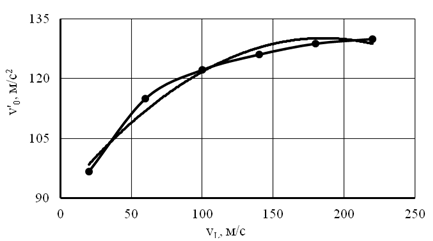
Рис. 3. Зависимость
начального градиента скорости v’(0)
от финишной скорости v(L): 1 – численное
моделирование; 2 – уравнение квадратичной регрессии
Однако нахождение каждого нового
значения функции v’0
требует
численного интегрирования системы (1,2), т. е. достаточно трудоемко. Поэтому
желательно использовать более быстрый метод, чем дихотомия. В [5,6] предложено
еще несколько методов. Нами был протестирован аналог метода Ньютона. Однако он
дал приблизительно такие же результаты, как и метод дихотомии (табл. 1).
В связи со значительным числом
прогона модели (более 300 раз, отсюда число численного интегрирования более
3000) при ее реальном использовании на производстве необходима минимизации
числа итераций. Для дальнейшего анализа при моделировании был построен график
искомой функции
References
1. Kalabin A.L. Elongatsionnoe techenie strui rastvorov i rasplavov polimerov. Tver': TvGTU, 2011. 144 s.
2. Zyabitskii A. Teoreticheskie osnovy formovaniya volokna. M.: Khimiya, 1979. 504 c.
3. Kalabin A.L., Kernitskii A.V., Pakshver E.A. Programmnaya sistema predproektnykh issledovanii tekhnologicheskikh protsessov formovaniya khimicheskikh volokon // Programmnye produkty i sistemy. 2008. № 1. C. 34-36.
4. Kalabin, A.L. Programmnaya sistema issledovanii dinamiki tekhnologicheskikh protsessov formovaniya khimicheskikh volokon / A.L. Kalabin, E.V. Udalov, A.R. Khabarov // Programmnye produkty i sistemy. 2015. Vyp. 1. S. 139-144.
5. Kalitkin N.N. Chislennye metody: uch. posobie. 2-e izd., ispr. SPb.: BKhV-Peterburg, 2011. 592 s.: il.
6. Bakhvalov N.S., Zhidkov N.P., Kobel'kov G.M. Chislennye metody. M.: Laboratoriya Bazovykh Znanii, 2000. 624 s.
7. Kalabin A.L., Udalov E.V. Dynamic characteristics of filament melt-spinning // Fibre Chemistry. 2013. 44. № 6. r. 356-360.
8. Kalabin A.L., Udalov E.V. Modeling the Dynamics of the Aerodynamic Forming of Fibers // Fibre Chemistry. 2014. 46. № 1. p. 10-15.
Link to this article
You can simply select and copy link from below text field.
|





